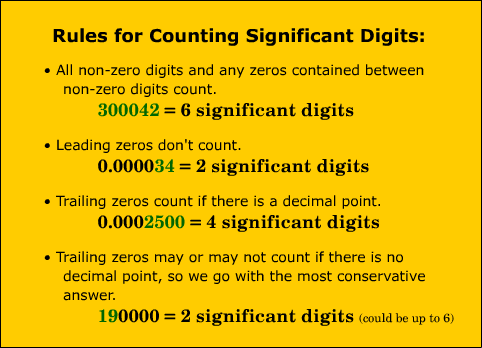
What defines a S.F.?
" The significant figures of a number are those digits that carry meaning contributing to its decision".
There are basic ground rules for S.F.s
1.Digits with no zeros are always significant. Example: 2.4 (2 s.f.), 5281 (4 s.f.)
2.All zeros after the decimal point are significant. Example: 0.500 (3 s.f.), 4.0 (2 s.f.)
3. Zeros that are in between two other significant digits are significant. Example: 7.06 (3 s.f.), 10.09 (4 s.f.)
4. Zeros used only for spacing decimal points are not significant.Example: 0.003 (1 s.f.), 0.020 (2 s.f.)
Calculation of physical data
1. Addition and subtraction: The final value has the same number of decimal places/ place value as the least precise measurement. Example: 44.1 + 8.002 + 0.93 = 53.0(1 d.p.)
(1 d.p.) (3 d.p.) (2 d.p.)
2.Multiplication and division: The product/quotient has the same number of significant figures as the number with the least number of significant figures. Example: 21.3 X 9.800 = 209 / 100.2 ÷ 0.50 = 2.0 X 100
(3 s.f.) (4 s.f.) (3 s.f.) (4 s.f.) (2 s.f.) ( 2 s.f. )
3.Average: The final value has the same number of decimal place/ place value as the least precise measurement
Example: velocity = (45.0 + 48.21 + 47.024) ÷ 3 = 46.7 km/h (1 d.p.)
(1 d.p.) (2 d.p.) (3 d.p.)
4.Constant: The number of decimal place / place value of a constant is not calculated in a calculation
Example: 0.5 m v2 = 0.5 X 1.55 X 2.0 X 2.0= 3.1 J (2 s.f.)
(3 s.f.) ( 2 s.f./ squared)
NOTE: 120( 3 place values)
120 has lesser precise measurement than 121.123
images and videos from google and youtube respectively